
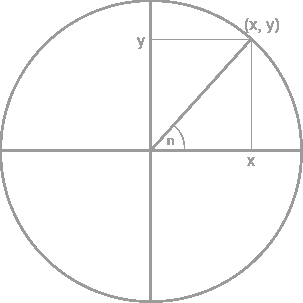

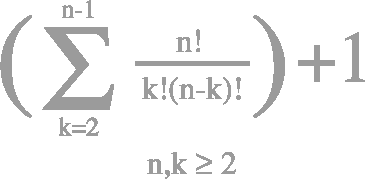




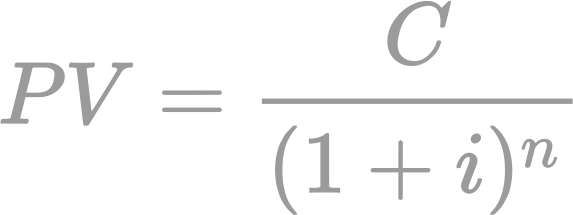
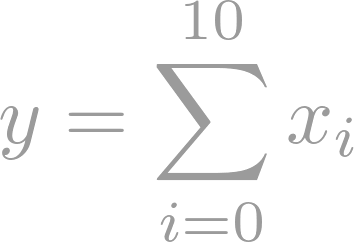
We consider the limitations of the Riemann integral, and show that it is necessary to develop a precise mathematical notion of `length' and `area' in order to overcome them. Thus we develop the more abstract concept of measure, and use it to construct the more Lebesgue integral, and to investigate its properties. Finally we look at the role played by measure and Lebesgue integration in modern probability theory. This course is intended to develop the ability of students to work with abstract ideas.